GATE Electrical 2022 | GA Question: 2

Question

In a $500\;m$ race, $P$ and $Q$ have speeds in the ratio of $3 : 4$. $Q$ starts the race when $P$ has already covered $140\; m$.

What is the distance between $P$ and $Q$ (in $m$) when $P$ wins the race?

• A. $20$
• B. $40$
• C. $60$
• D. $140$

Answer 1

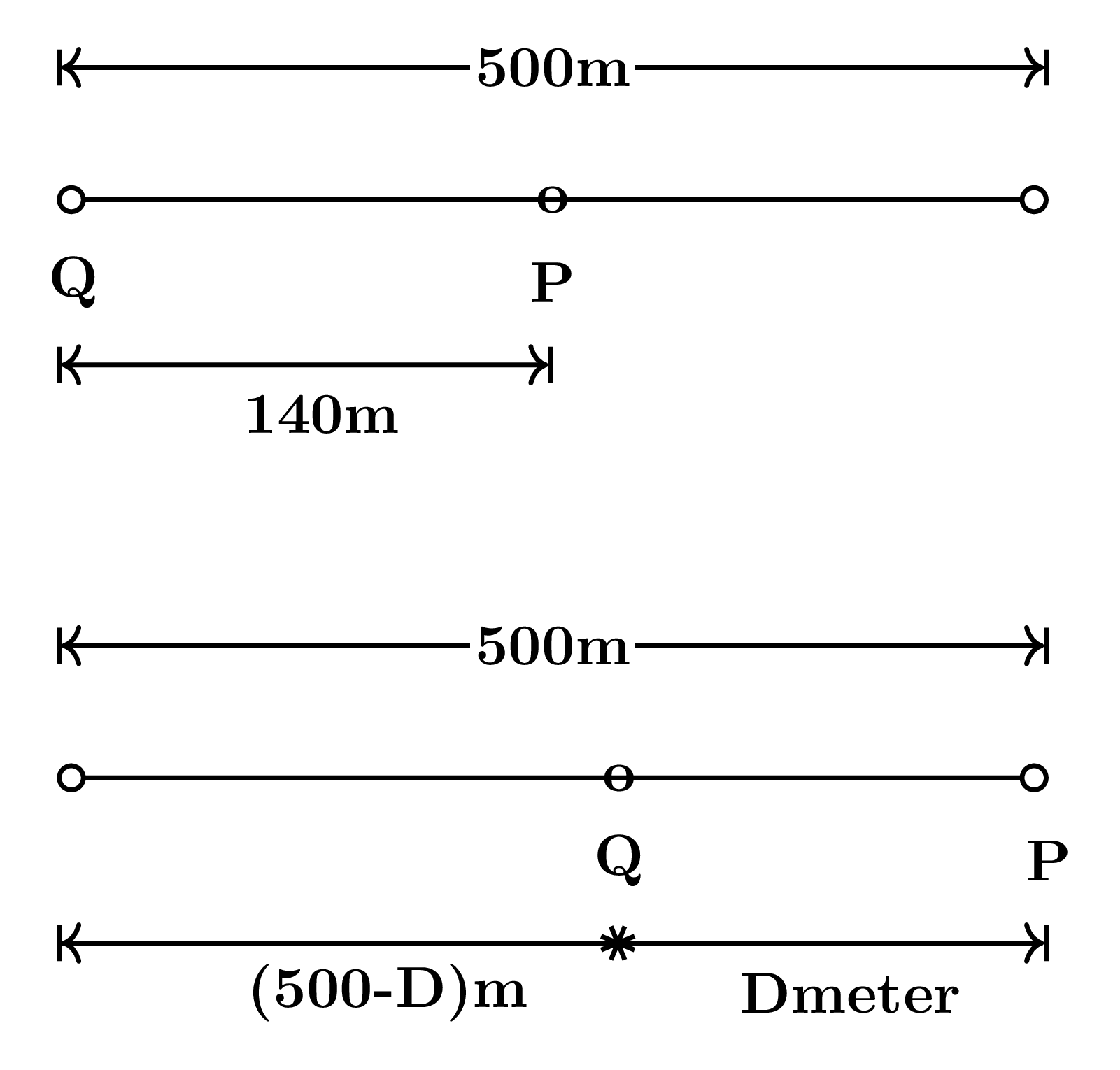
Let’s first draw the diagram for a better understanding.

Let the distance between $\text{P},$ and $\text{Q}$ be $\text{‘D’}$ meter.

Given that, $\text{S}_{\text{P}}:\text{S}_{\text{Q}} = 3:4 \Rightarrow {\color{Blue}{\boxed{\frac{\text{S}_{\text{P}}}{\text{S}_{\text{Q}}} = \frac{3}{4}}}}$

We know that,  ${\color{Green}{\text{Speed} = \dfrac{\text{Distance}}{\text{Time}} \Rightarrow \text{Speed} \propto \text{Distance (Time constant)}}}$

$\implies {\color{Purple}{\boxed{\frac{\text{S}_{1}}{\text{S}_{2}} = \frac{\text{D}_{1}}{\text{D}_{2}}}}}$

Now, $\dfrac{3}{4}  = \dfrac{500-140}{500-\text{D}}$

$\Rightarrow \dfrac{3}{4}  = \dfrac{360}{500-\text{D}}$

$\Rightarrow 3(500-\text{D}) = 4 \times 360$

$\Rightarrow 1500-3\text{D} = 1440$

$\Rightarrow 3\text{D} = 60$

$\Rightarrow {\color {Blue}{\boxed{\text{D = 20}}}}$

$\therefore$ The distance between $\text{P}$ and $\text{Q},$ when $\text{P}$ wins the race is $20\;\text{meters}.$

Correct Answer $:\text{A}$